The Lebesgue-Stieltjes Integral : Summary

Firstly, something on the pronunciation –:).Lebesgue Stieltjes is pronounced as Le-BECK Steel-ye. The former being a French mathematician and the latter being a Dutch mathematician and the integral being named after their outstanding contribution to the field of analysis. I came across Stieltjes integral for the first time in Marek Capinski’s book on Probability. It was introduced in relation to measure decomposition theorems. The treatment in Marek Capinski’s book is very concise and hence I really did not understand the significance of Lebesgue Stieltjes integral. Also I came across this reference to this integral while I was studying Ito’s integral a few years back. Back then, I had no clue about Lebesgue Stieltjes integral and had never bothered to check its limitations in describing Brownian Motion. All I cared that it was somehow useless for describing Brownian motion. My limited understanding became a handicap in understanding measure decompositions. So, finally I had to get out of limbo and slog through to understand the integral. I picked up this book which looked like an accessible introduction to the integral, which in the hindsight appears the perfect choice. Let me try to summarize the contents of the book.
The first three chapters of the book cover the prerequisites needed to understand the Lebesgue-Stieltjes integral. Basic terms like supremum, infimum, Cardinality, Topology of R are covered in the Chapter 1. Chapter 2 talks about monotone sequences, monotone functions and explores their properties. It subsequently introduces the concept of bounded variation and absolute continuity. Absolute continuous functions are a subset of continuous functions. A few theorems are stated relating to functions which have bounded variation. Chapter 3 gives a basic introduction to Riemann integral and cites the Dirichlet function as an example where Riemann integral breaks down. Not only is the function not Riemann integrable, it reveals a bigger problem with Riemann integral. If we consider a sequence of functions that are Riemann integrable, then we might not be certain that the limiting function is Riemann integrable.
Chapter 4 is the meat of the book. The approach taken by this book is the step function approach. Instead of taking measure theory approach, the book takes a step function approach. As is found in most books which avoid measure theory, the step function approach is an easier way to understanding Lebesgue Stieltjes and Lebesgue integral. The following term are introduced :

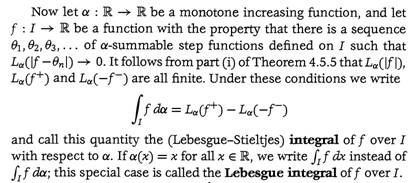
Subsequently Lebesgue-Stieltjes integral is defined as follows. Let me try to put in simple words( which is always a challenge than writing an equation). One starts off with sequence of step functions, applies certain criteria like finite measure and then creates a sequence of these functions called alpha summable. The key idea is this :
If you have a function and you want to integrate the function over Interval I with respect to alpha measure, You create a set of all possible alpha summable functions and in each case the alpha summable function is always greater than the function that we are interested in integrating. So, instead of integrating the complicated function , we are integrating all possible alphas summable functions over the same interval. Obviously the latter is easier to do as they are all internally a combination of step functions. Once you find the integral of all these alpha summable functions, you take the minimum of those values, or to use the right word, infimum of all those values, what you get is the Lebesgue-Stieltjes integral with respect to alpha measure. (That was mouthful!) . To see it with math symbols( which is so elegant) it is as follows :
The chapter ends with a discussion on the differences between Riemann and Lebesgue integral. Basically the thing to be understood is that Lebesgue integral of a function is computed by a set of approximating functions which are much more generic than the step functions used in the Riemann sense. This coupled with the fact that the measure itself is generic makes Lebesgue integral applicable to a mind boggling set of functions. However as the author rightly points, Lebesgue integral’s true power is visible in Convergence theorems which help in computing integrals of very complicated functions over complicated sets –:)( For example Dirichlet function). There is also a nice example of a function which is Riemann integrable and not Lebesgue integrable. One key requirement for any function to be Lebesgue integrable is that the integral must be absolutely convergent.
Chapter 5 deals with the properties of Lebesgue-Stieltjes integral. Integrability of f+, f-, |f| are explored. The section on null sets and null functions is very tedious. For someone who is exposed to measure theory where sets with measure 0 and null sets are explored at the very beginning, the treatment in this book looks very circuitous. All the proofs in this section of the chapter can be massively simplified by using set theory concepts and using measure theory. So, even though step functions can be used to interpret lebesgue integrals easily, there is a flip side to it. Things like null sets becomes very challenging to understand. The chapter then states the important convergence theorems like Monotone Convergence Theorem, Fatou’s Lemma, Dominated Convergence Theorem, Beppo-Levi’s theorem. All of these are merely stated and no proof is given. The reader should appreciate that these theorems are the main reason that Lebesgue theory became massively popular. Dominated Convergence theorem is a very broad sufficient condition ( Note: It is not a necessary condition). So, these theorems can be used to approximate complicated integrals by sequence of functions and then integral and limit can be swapped to compute the value of the integral. I found the last section of this chapter to be very interesting. It talks about extending theory in two directions 1) by allowing integration over sets than intervals 2) allowing the alpha measure to be a function of bounded variation. The second direction is about splitting a alpha measure function with bounded variation in to two monotonic functions. This discussion is closely related to measure decomposition. A related topic is Jordan decomposition. I came across a nice way of describing measure decomposition in a blog “A Mind for Madness”.Its worth a read
Chapter 6 is useful from a computational point of view. A laundry list of theorems is stated that are useful for evaluating Lebesgue-Stieltjes integral. The chapter also explores change of variable and integration by parts in L-S world.A few examples are cited where to evaluate an integral, it is better to differentiate the entire equation and then apply integration on the differential. Most of them are computational tricks rather than any conceptual principles. Chapter 7 extends the Lebesgue Stieltjes integral to multidimensional case. While dealing with double integral , the order of integration does matter, meaning it is possible that if you integrate first with respect to x and then with respect to y, you get a totally different result than if you had integrated first with respect to y and then x. The condition under which the order of integration does not matter is when the function involved in the double integral sign is absolutely convergent with respect to x and y. Fubini’s theorem is just this. It specifies conditions under which the order of integration does not matter. In terms of the usual measure theory perspective , Fubini’s theorem is nothing but a condition on the integrabilty of projection function on the various measures involved. If these projections are measurable functions, they Fubini’s theorem says that order doesn’t matter.
Chapter 8 offers a crash course in functional analysis. Beyond a certain point, one would like some structure in various Lebesgue measurable integrals. The chapter gives some basic definitions of vector spaces, normed vector spaces, norm, linear dependence, function spaces, Cauchy sequences etc. It then shows that the space of continuous functions cannot be equipped with norm based on Riemann integral as it creates a lot of problems. Dirichlet function is one where the sequence of functions are Riemann integrable but the limiting function escapes from this space where norm is defined based on Riemann Integral. Hence there is a case for a better norm and that norm turns out to be based on Lebesgue integral. There is a small wrinkle with using Lebesgue integral as one of the definitions of norm is violated. If you take a function which is 0 almost everywhere, the norm turns out to be 0 but the function as such is not 0. Spaces such as these have a specific name , “semi normed vector spaces”. It can be shown that semi normed vector spaces can be partitioned in to equivalence classes based on almost everywhere property and subsequently , this space is a nice mathematical space meaning, it is a complete space. Every Cauchy sequence of functions based on L1 norm converges to a function in the same space. Similarly LP spaces are also complete normed vector spaces. Any space which is a complete normed vector space is a Banach space and hence all LP spaces are Banach spaces.
Amongst all LP spaces , the space relating to p = 2 offers an unique opportunity to define inner product space and thus enabling one to marry the concepts of orthogonality/projection etc with Lebesgue measurable functions. This space is referred to as Hilbert Space, in recognition to the remarkable contribution of David Hilbert, the German mathematician who developed a broad range of fundamental ideas in many areas, including invariant theory and the axiomatization of geometry. Hilbert Spaces are pivotal to functional analysis and are discussed in Chapter 9. It talks about application of properties of Hilbert Spaces to Fourier Series and PDEs. Obviously you get only a 1000 ft view of this stuff and you have to refer other texts if the applied math interests you in a specific area. From a math fin perspective though, viewing Fourier series from Hilbert space is vital as many option pricing problems are solved using Fourier Series applications.
Chapter 10 is a cautionary note from the authors that all is not rosy with Lebesgue Stieltjes integral as it is valid only for absolutely convergent integrals. There is a whole host of conditionally convergent integrals which Lebesgue cannot handle. As a cue to an interested reader, the authors show developments post Lebesgue like Denjoy-Perron integrals, Henstock-Kurzweil integrals , thus ending with a opinion that perfect integration method is still an elusive dream.
I personally think that to integrate, you must be a fox rather than a hedgehog. Depending on the context, one must use a specific method and get done with the problem. An all encompassing integration method is like a hedgehog , and to me it appears that such a hedgehog has become an extinct species and chances of appearing is a remote possibility.
 Takeaway:
Takeaway:
This book provides an easy access to Lebesgue-Stieltjes via step function approach. One does not need to know measure theory to understand the book; however exposure to measure makes it an easy read. The highlight of the book is that it has a lot of examples that show the motivation behind Lebesgue-Stieltjes integral.